How accurate is FEA and CFD simulation?
A question I have been asked throughout the years when presenting Finite Element Analysis (FEA) and Computational Fluid Dynamics (CFD) to many companies is: How accurate is FEA and CFD simulation? While the question seems simple, the answer is not. In properly answering the question, I always look at who is asking the question and why. In this blog post, I will try to answer this question from different perspectives.
For a CAD designer with no prior exposure to FEA and CFD, this is a natural question to ask. Numerical methods go back a long time and have been used to approximate solutions to problems that cannot be solved precisely. Since the 1950s, when computers became available for the heavy number-crunching of FEA and CFD, both these methods have been researched extensively; many thousands of books and research papers published; and the methodologies continuously improved and algorithms refined. FEA and CFD are sound reliable methods.
Within the documents included in our SOLIDWORKS FEA and CFD Simulation products, there are validation problems that compare the numerical solution to selected problems with a closed-form analytical solution, established experimental results or solutions from other resources.
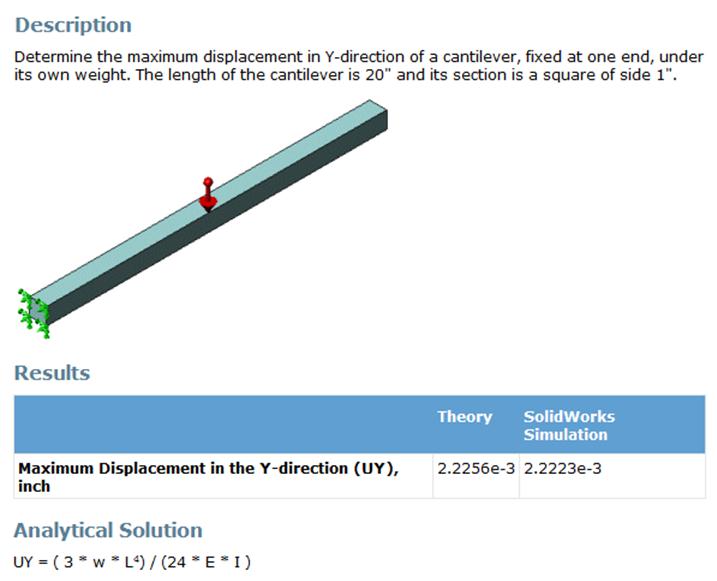
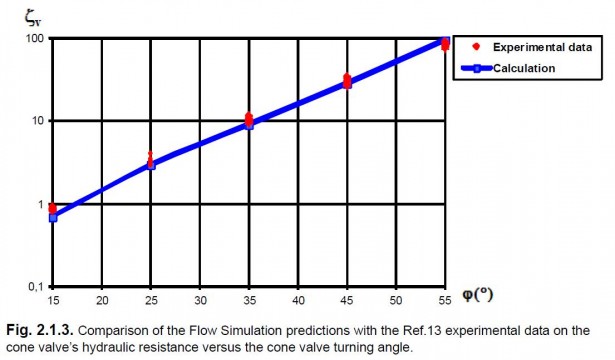
For a power user concerned with accuracy, the discussion starts about continuum discretization and meshing. Types of elements in FEA, such as solids, shells and beams or 2D elements like plane-stress, plane-strain and axi-symmetric, our two FEA meshing algorithms (standard & curvature-based), various mesh controls and compatible vs. incompatible mesh methodologies typically come up.
An ideal numerical solution is one that is mesh-independent. After all, meshing is an approximation of a continuum into a discretized finite model. It is basically math and not physics. While complete mesh-independency may never be achieved, as engineers, we seek the converged solution within acceptable approximations and tolerances. Adaptive meshing, in which the program adjusts the mesh during the calculation by looking at gradients of the parameter under consideration are of particular interest to power users.
The question of convergence also comes up when solving nonlinear problems in FEA, be it material nonlinearities, such as structural behavior beyond yielding or using a nonlinear stress-strain curve, geometric nonlinearities as with large deflections or general contact. In CFD, where we solve the coupled partial differential equations of Navier-Stokes, convergence graphs are plotted for selected parameters.
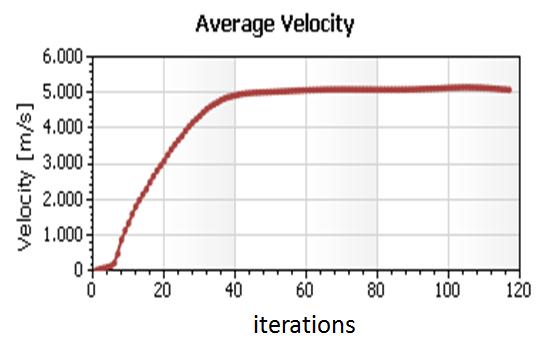
Sometimes, the question of accuracy is asked by customers and prospects not based on the methodologies and/or program implementation and capabilities, but with a particular problem in mind. First make sure that the type of simulation you run corresponds to the mode of failure you are observing or expecting in reality. A static simulation examining strength does not tell you much about a real world fatigue failure that examines life under cyclic loading. A linear analysis is only good as long as the underlying linearity assumptions are satisfied. When you go beyond material yield strength anywhere in your design, you will have a re-distribution of stress which can only be captured by a nonlinear analysis. Sometimes, the distinctions are slightly more subtle. High-cycle fatigue uses a different methodology than low-cycle fatigue, so depending on the type of problem you have to watch for these nuances. If you do not know which failure mode you have to pursue, you either should simulate all different modes of failure or do some experiments in the lab to gain a better understanding of which failure modes to rule in or out.
When trying to replicate experimental data or actual response in the field with simulation, remember that the source of potential discrepancies is often in the setups. As an example, consider this part acting as a simple cantilever. Depending on the boundary condition and the relative stiffness of the part versus its supporting structure, you will get different results.
In addition to boundary conditions, material properties and the respective material model utilized to represent it can be another consideration. The more accurate quantitative results you are seeking, the more careful you should be with the setup of your model and interpretation of results and the nuances involved. Whereas if you are looking for a more qualitative understanding of the behavior at the design stage, you could be more open-minded to the approximations involved.
The question of accuracy should be addressed in context. FEA and CFD are extremely powerful tools that can be used by Product engineers very effectively. The earlier you start using simulation in your design the more effective it will be to help you understand behaviors and trends. You can see where the critical regions are and what may need more attention for a more detailed analysis. Using simulation at later stages can become somewhat more involved depending on the situation, but even then this is one of the most effective tools that we engineers have at our disposal. Let’s try to use it early, often, and accurately.
Click here to watch videos, read white papers and hear from customers about how SOLIDWORKS provides the most unique, complete and integrated simulation solutions from finite element analysis (FEA) and computational fluid dynamics (CFD) to plastics and sustainable design with the SOLIDWORKS Simulation solution.








